This square is divided into 36 smaller squares.
Amanda Montañez
If you put several of these small squares together, you can form larger squares. You can see two such squares here as an example:
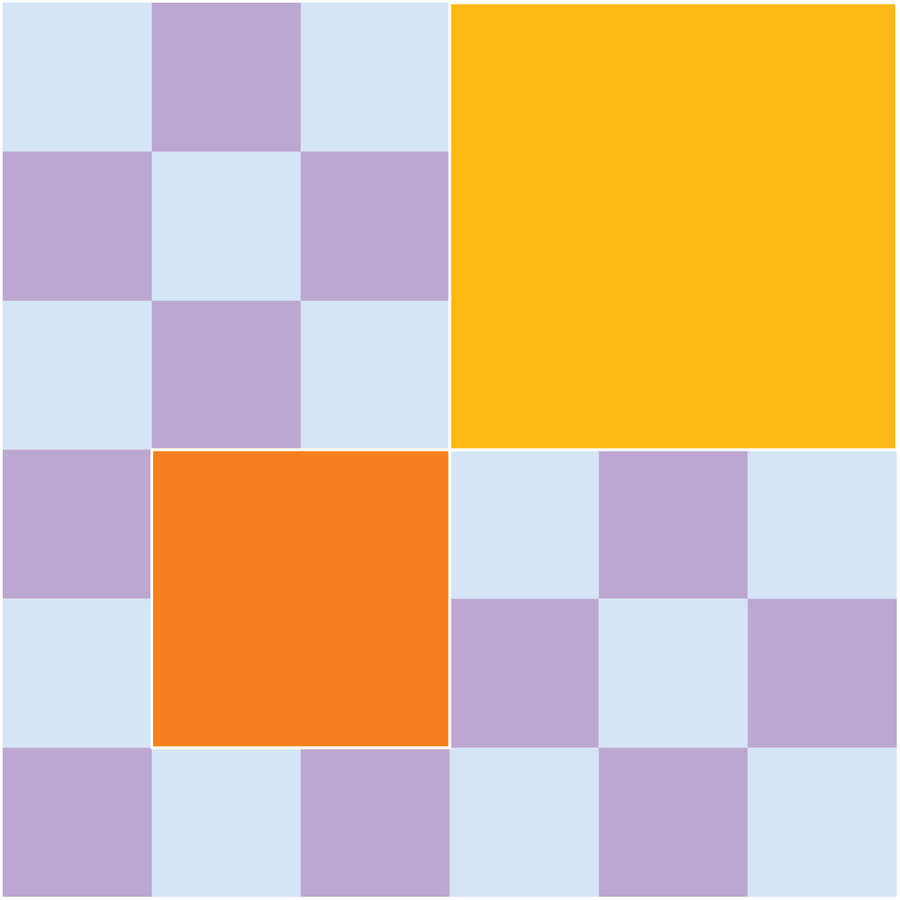
Amanda Montanez
How many squares are there to discover in total?
There are 91 squares in total. Because the big square has a side length of 6, there are six different square sizes: squares with lengths 1, 2, 3, 4, 5 and 6.
You can find 36 squares with length 1, 25 squares with length 2, 16 squares with length 3, nine squares with length 4, four squares with length 5 and one square with length 6. In total, there are 36 + 25 + 16 + 9 + 4 + 1 = 91 squares.
In general, the total number of squares, represented by A, in a similarly divided square with side length n can be written as:
A = n2 + (n – 1)2 + (n – 2)2 + (n – 3)2 ... + (n – n)2
We’d love to hear from you! E-mail us at games@sciam.com to share your experience.
This puzzle originally appeared in Spektrum der Wissenschaft and was reproduced with permission.